README_EN.md

1401. Circle and Rectangle Overlapping

中文文档

Description

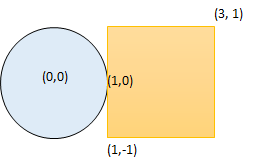
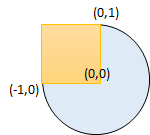You are given a circle represented as (radius, xCenter, yCenter) and an axis-aligned rectangle represented as (x1, y1, x2, y2), where (x1, y1) are the coordinates of the bottom-left corner, and (x2, y2) are the coordinates of the top-right corner of the rectangle.

Return true if the circle and rectangle are overlapped otherwise return false. In other words, check if there is any point (xi, yi) that belongs to the circle and the rectangle at the same time.

 

Example 1:

Input: radius = 1, xCenter = 0, yCenter = 0, x1 = 1, y1 = -1, x2 = 3, y2 = 1
Output: true
Explanation: Circle and rectangle share the point (1,0).

Example 2:

Input: radius = 1, xCenter = 1, yCenter = 1, x1 = 1, y1 = -3, x2 = 2, y2 = -1
Output: false

Example 3:

Input: radius = 1, xCenter = 0, yCenter = 0, x1 = -1, y1 = 0, x2 = 0, y2 = 1
Output: true

 

Constraints:

• 1 <= radius <= 2000
• -104 <= xCenter, yCenter <= 104
• -104 <= x1 < x2 <= 104
• -104 <= y1 < y2 <= 104

Solutions

Solution 1: Mathematics

For a point $(x,y)$, its shortest distance to the center of the circle $(xCenter,yCenter)$ is $\sqrt{(x-xCenter{)}^2+(y-yCenter{)}^2}$. If this distance is less than or equal to the radius $radius$, then this point is within the circle (including the boundary).

For points within the rectangle (including the boundary), their x-coordinates $x$ satisfy $x_1\le x\le x_2$, and their y-coordinates $y$ satisfy $y_1\le y\le y_2$. To determine whether the circle and rectangle overlap, we need to find a point $(x,y)$ within the rectangle such that $a=|x-xCenter|$ and $b=|y-yCenter|$ are minimized. If $a^2+b^2\le radiu{s}^2$, then the circle and rectangle overlap.

Therefore, the problem is transformed into finding the minimum value of $a=|x-xCenter|$ when $x\in [x_1,x_2]$, and the minimum value of $b=|y-yCenter|$ when $y\in [y_1,y_2]$.

For $x\in [x_1,x_2]$:

• If $x_1\le xCenter\le x_2$, then the minimum value of $|x-xCenter|$ is $0$;
• If $xCenter<x_1$, then the minimum value of $|x-xCenter|$ is $x_1-xCenter$;
• If $xCenter>x_2$, then the minimum value of $|x-xCenter|$ is $xCenter-x_2$.

Similarly, we can find the minimum value of $|y-yCenter|$ when $y\in [y_1,y_2]$. We can use a function $f(i,j,k)$ to handle the above situations.

That is, $a=f(x_1,x_2,xCenter)$, $b=f(y_1,y_2,yCenter)$. If $a^2+b^2\le radiu{s}^2$, then the circle and rectangle overlap.

Python3

class Solution:
    def checkOverlap(
        self,
        radius: int,
        xCenter: int,
        yCenter: int,
        x1: int,
        y1: int,
        x2: int,
        y2: int,
    ) -> bool:
        def f(i: int, j: int, k: int) -> int:
            if i <= k <= j:
                return 0
            return i - k if k < i else k - j

        a = f(x1, x2, xCenter)
        b = f(y1, y2, yCenter)
        return a * a + b * b <= radius * radius

Java

class Solution {
    public boolean checkOverlap(
        int radius, int xCenter, int yCenter, int x1, int y1, int x2, int y2) {
        int a = f(x1, x2, xCenter);
        int b = f(y1, y2, yCenter);
        return a * a + b * b <= radius * radius;
    }

    private int f(int i, int j, int k) {
        if (i <= k && k <= j) {
            return 0;
        }
        return k < i ? i - k : k - j;
    }
}

C++

class Solution {
public:
    bool checkOverlap(int radius, int xCenter, int yCenter, int x1, int y1, int x2, int y2) {
        auto f = [](int i, int j, int k) -> int {
            if (i <= k && k <= j) {
                return 0;
            }
            return k < i ? i - k : k - j;
        };
        int a = f(x1, x2, xCenter);
        int b = f(y1, y2, yCenter);
        return a * a + b * b <= radius * radius;
    }
};

Go

func checkOverlap(radius int, xCenter int, yCenter int, x1 int, y1 int, x2 int, y2 int) bool {
	f := func(i, j, k int) int {
		if i <= k && k <= j {
			return 0
		}
		if k < i {
			return i - k
		}
		return k - j
	}
	a := f(x1, x2, xCenter)
	b := f(y1, y2, yCenter)
	return a*a+b*b <= radius*radius
}

TypeScript

function checkOverlap(
    radius: number,
    xCenter: number,
    yCenter: number,
    x1: number,
    y1: number,
    x2: number,
    y2: number,
): boolean {
    const f = (i: number, j: number, k: number) => {
        if (i <= k && k <= j) {
            return 0;
        }
        return k < i ? i - k : k - j;
    };
    const a = f(x1, x2, xCenter);
    const b = f(y1, y2, yCenter);
    return a * a + b * b <= radius * radius;
}

...